Question 1
Define: elastic deformation, plastic deformation and elastic limit
Easy
Mark as Complete
Mark Scheme
Question 2
The force–extension graph for a spring is shown.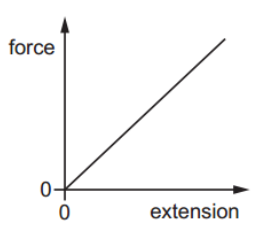 What represents the work done to extend the spring?
What represents the work done to extend the spring?
A. The area under the graph.
B. The gradient of the graph.
C. The reciprocal of the gradient of the graph.
D. Twice the area under the graph.
Easy
Mark as Complete
Mark Scheme
Question 3
The spring constants of four springs are determined by plotting the following graphs of force F against extension x:
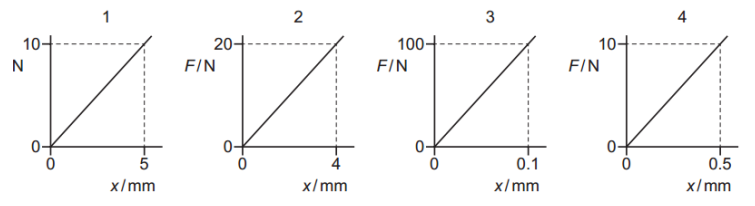
Which order of the graphs shows decreasing spring constants?
A. 2 → 1 → 3 → 4.
B. 3 → 4 → 2 → 1.
C. 4 → 2 → 1 → 3.
D. 4 → 3 → 2 → 1.
Easy
Mark as Complete
Mark Scheme
Question 4
The graph shows how the length of a spring varies with the force applied to it. The spring has unstretched length `L_0`. When a force is applied, the spring has length `L_1`.
The spring has unstretched length `L_0`. When a force is applied, the spring has length `L_1`.
What is the work done in stretching the spring to length `L_1`?
Easy
Mark as Complete
Mark Scheme
Question 5
What is an example of plastic deformation?
A. A rubber ball is momentarily compressed every time it hits the ground.
B. A spoon stirring some coffee in a ceramic mug hits its surface and makes a clinking sound.
C. A toolbox is left on a horizontal plank. When the toolbox is removed, the plank is no longer straight.
D. The spring in some bathroom weighing scales is compressed by a person standing on the scales.
Medium
Mark as Complete
Mark Scheme
Question 6
Which statement describes what is meant by the plastic deformation of a material?
A. It always obeys Hooke’s law.
B. It does not return to its original length when the extending force is removed.
C. It never obeys Hooke’s law.
D. It returns to its original length when the extending force is removed.
Easy
Mark as Complete
Mark Scheme
Question 7
Which statement about elastic and plastic deformation must be correct?
A. Elastic deformation and plastic deformation are proportional to the applied force.
B. Elastic deformation and plastic deformation cause no change in volume.
C. Elastic deformation causes heating of the material but plastic deformation does not.
D. Elastic deformation is reversible but plastic deformation is not.
Medium
Mark as Complete
Mark Scheme
Question 8
Forces are applied to the ends of a rod so that its length increases. The variation with force F of the extension of the rod is shown. The point P is the elastic limit.
The point P is the elastic limit.
Which shaded area represents the work done during the plastic deformation of the rod?
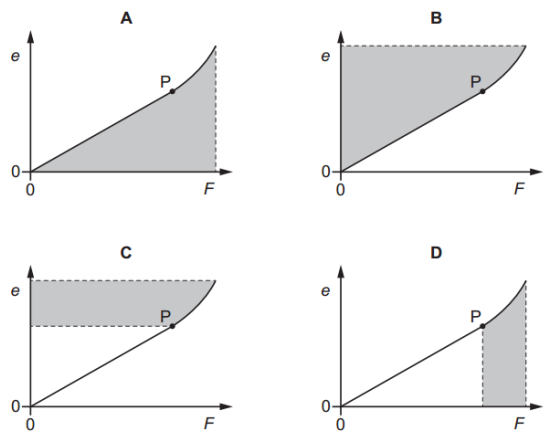
Medium
Mark as Complete
Mark Scheme
Question 9
A spring is suspended from a fixed point at one end. The spring is extended by a vertical force applied to the other end. The variation of the applied force with the length L of the spring is shown in figure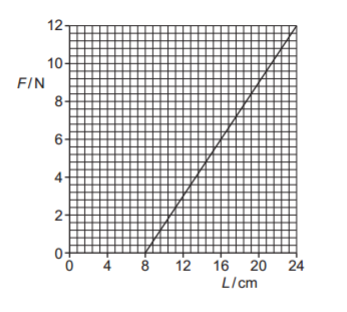 a. State the name of the law that gives the relationship between the force and the extension.
a. State the name of the law that gives the relationship between the force and the extension.
b. Determine the spring constant, in N.m⁻¹.
c. Determine the elastic potential energy when F = 6 N.
Medium
Mark as Complete
Mark Scheme
Question 10
A spring is suspended from a fixed point at one end. A vertical force is applied to the other end, as shown in figure.
The variation of the applied force with the length L of the spring is shown in figure.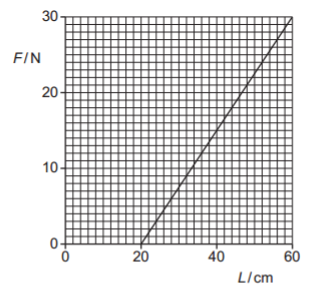 a. Determine the spring constant of the spring.
a. Determine the spring constant of the spring.
b. Determine the elastic potential energy in the spring when the applied force is 15 N.
Medium
Mark as Complete
Mark Scheme
Question 1
Define: elastic deformation, plastic deformation and elastic limit
Elastic deformation: Elastic deformation is the temporary change in shape or size of a material when a force is applied. The material returns to its original shape once the force is removed.
Plastic deformation: Plastic deformation is the permanent change in shape of a material when the applied force exceeds the elastic limit. The material does not return to its original shape when the force is removed.
Elastic limit: The elastic limit is the maximum force or stress that can be applied to a material without causing permanent deformation
Question 2
The force–extension graph for a spring is shown. What represents the work done to extend the spring?
What represents the work done to extend the spring?
A. The area under the graph.
B. The gradient of the graph.
C. The reciprocal of the gradient of the graph.
D. Twice the area under the graph.
Answer: A
A. Correct:
The work done on a spring is the energy stored in it when extended:
`W = 1/2 F x`
This is the area under the force-extension graph, which is a triangle.
B. Incorrect: The gradient represents the spring constant kk, not the work.
C. Incorrect: It represents the inverse of stiffness.
D. Incorrect: That would give Fx, which overestimates the actual work done by a factor of 2.
Question 3
The spring constants of four springs are determined by plotting the following graphs of force F against extension x:

Which order of the graphs shows decreasing spring constants?
A. 2 → 1 → 3 → 4.
B. 3 → 4 → 2 → 1.
C. 4 → 2 → 1 → 3.
D. 4 → 3 → 2 → 1.
Answer: B
To find the spring constant from each graph, we calculate the gradient of the line, which is the slope:
`"Slope"=(DeltaF)/(Deltax)=k`
Graph 1:
F = 10 N, x=5 mm = 0.005 m
`k = 10 / 0.005 = 2000 " " N.m^-1`
Graph 2:
F = 20 N, x = 4 mm = 0.004 m
`k = 20 / 0.004 = 5000 " " "N.m^-1`
Graph 3:
F = 100 N, x = 0.1 mm = 0.0001 m
`k = 100 / 0.0001 = 1000000 " "N.m^-1`
Graph 4:
Question 4
The graph shows how the length of a spring varies with the force applied to it. The spring has unstretched length `L_0`. When a force is applied, the spring has length `L_1`.
The spring has unstretched length `L_0`. When a force is applied, the spring has length `L_1`.
What is the work done in stretching the spring to length `L_1`?
For a spring that follows Hooke’s Law, the work done to stretch it is equal to the area under the force-extension graph:
`W = 1/2xx Fxx (L_1 - L_0)`
Question 5
What is an example of plastic deformation?
A. A rubber ball is momentarily compressed every time it hits the ground.
B. A spoon stirring some coffee in a ceramic mug hits its surface and makes a clinking sound.
C. A toolbox is left on a horizontal plank. When the toolbox is removed, the plank is no longer straight.
D. The spring in some bathroom weighing scales is compressed by a person standing on the scales.
Answer: C
A. Incorrect: The compression of a rubber ball is temporary. Once the ball rebounds, it returns to its original shape. This is an example of elastic deformation, not plastic.
B. Incorrect: The clinking sound from a spoon hitting the mug is due to vibration and contact, not deformation. There's no shape change involved, so this is irrelevant to both elastic and plastic deformation.
C. Correct: This is a clear example of plastic deformation. When the plank bends and does not return to its original straight shape after the toolbox is removed, it has undergone permanent deformation.
D. Incorrect: The spring in the weighing scale returns to its original shape when the person steps off. This is again elastic deformation, which is reversible.
Question 6
Which statement describes what is meant by the plastic deformation of a material?
A. It always obeys Hooke’s law.
B. It does not return to its original length when the extending force is removed.
C. It never obeys Hooke’s law.
D. It returns to its original length when the extending force is removed.
Answer: B
A. Incorrect: Hooke’s law is only valid within the elastic limit, where force is proportional to extension. Plastic deformation occurs after Hooke’s law no longer applies.
B. Correct: Plastic deformation occurs when a material is stretched beyond its elastic limit, resulting in a permanent change in shape or length. Once the force is removed, the material does not return to its original length.
C. Incorrect: The material may obey Hooke’s law in the elastic region before plastic deformation begins.
D. Incorrect: This is the definition of elastic deformation, not plastic deformation.
Question 7
Which statement about elastic and plastic deformation must be correct?
A. Elastic deformation and plastic deformation are proportional to the applied force.
B. Elastic deformation and plastic deformation cause no change in volume.
C. Elastic deformation causes heating of the material but plastic deformation does not.
D. Elastic deformation is reversible but plastic deformation is not.
Answer: D
A. Incorrect: Only elastic deformation is proportional to force (within the elastic limit).
B. Incorrect: Some materials do change volume during plastic deformation (especially polymers or foams). The statement is too absolute to be correct.
C. Incorrect: Plastic deformation often leads to more internal energy dissipation (as heat) due to atomic movement. Elastic deformation involves stored energy, not heat.
D. Correct:
Elastic deformation:
When a material is deformed elastically, it returns to its original shape once the force is removed. This occurs within the elastic limit and follows Hooke’s Law.
Plastic deformation:
If the force exceeds the elastic limit, the material undergoes plastic deformation, meaning it permanently changes shape and does not return to its original form.
Question 8
Forces are applied to the ends of a rod so that its length increases. The variation with force F of the extension of the rod is shown. The point P is the elastic limit.
The point P is the elastic limit.
Which shaded area represents the work done during the plastic deformation of the rod?

Answer: C
A. Incorrect: Includes the area under the entire curve, both elastic and plastic regions.
B. Incorrect: Represents the area above the force-extension curve, which does not correspond to any physical work done.
C. Correct: Shows the area between the curve and the elastic limit line, i.e., the area under the curve beyond point P.
D. Incorrect: Shows only a vertical slice, which does not represent the area under the curve beyond P.
Question 9
A spring is suspended from a fixed point at one end. The spring is extended by a vertical force applied to the other end. The variation of the applied force with the length L of the spring is shown in figure a. State the name of the law that gives the relationship between the force and the extension.
a. State the name of the law that gives the relationship between the force and the extension.
b. Determine the spring constant, in N.m⁻¹.
c. Determine the elastic potential energy when F = 6 N.
a. Hooke's laws
b. From the graph:
When F = 12 N, L = 24 cm = 0.24 m
Unstretched length L0 = 8 cm = 0.08 m
Extension: x = 0.24 − 0.08 = 0.16 m
`k = F / x = 12 / 0.16 = 75 " N.m"^-1`
c. From the graph:
When F = 6 N, L = 16 cm = 0.16 m
Unstretched length L0 = 8 cm = 0.08 m
Extension: x = 0.16 − 0.08 = 0.08 m
`E = 1/2xx Fxx x = 1/2 xx6xx 0.08 = "0.24 J"`
Question 10
A spring is suspended from a fixed point at one end. A vertical force is applied to the other end, as shown in figure.
The variation of the applied force with the length L of the spring is shown in figure. a. Determine the spring constant of the spring.
a. Determine the spring constant of the spring.
b. Determine the elastic potential energy in the spring when the applied force is 15 N.
a. From the graph:
When F = 30 N, the total length L = 60 cm = 0.6 m
The natural length (unstretched length) L0 = 20 cm = 0.2 m
So, extension x = 0.6 − 0.2 = 0.4 m
`k = F / x = 30 / 0.40 = 75 " N"."m"^-1`
b. From the graph:
When F = 15 N, the length of the spring L = 40 cm = 0.4 m
So, extension x = 0.4 − 0.2 = 0.2 m
`E = 1/2xx kxx x^2 = 1/2 xx 75 xx (0.2)^2 = "1.5 J"`
Question 1
Define: elastic deformation, plastic deformation and elastic limit
Question 2
The force–extension graph for a spring is shown. What represents the work done to extend the spring?
What represents the work done to extend the spring?
A. The area under the graph.
B. The gradient of the graph.
C. The reciprocal of the gradient of the graph.
D. Twice the area under the graph.
Question 3
The spring constants of four springs are determined by plotting the following graphs of force F against extension x:

Which order of the graphs shows decreasing spring constants?
A. 2 → 1 → 3 → 4.
B. 3 → 4 → 2 → 1.
C. 4 → 2 → 1 → 3.
D. 4 → 3 → 2 → 1.
Question 4
The graph shows how the length of a spring varies with the force applied to it. The spring has unstretched length `L_0`. When a force is applied, the spring has length `L_1`.
The spring has unstretched length `L_0`. When a force is applied, the spring has length `L_1`.
What is the work done in stretching the spring to length `L_1`?
Question 5
What is an example of plastic deformation?
A. A rubber ball is momentarily compressed every time it hits the ground.
B. A spoon stirring some coffee in a ceramic mug hits its surface and makes a clinking sound.
C. A toolbox is left on a horizontal plank. When the toolbox is removed, the plank is no longer straight.
D. The spring in some bathroom weighing scales is compressed by a person standing on the scales.
Question 6
Which statement describes what is meant by the plastic deformation of a material?
A. It always obeys Hooke’s law.
B. It does not return to its original length when the extending force is removed.
C. It never obeys Hooke’s law.
D. It returns to its original length when the extending force is removed.
Question 7
Which statement about elastic and plastic deformation must be correct?
A. Elastic deformation and plastic deformation are proportional to the applied force.
B. Elastic deformation and plastic deformation cause no change in volume.
C. Elastic deformation causes heating of the material but plastic deformation does not.
D. Elastic deformation is reversible but plastic deformation is not.
Question 8
Forces are applied to the ends of a rod so that its length increases. The variation with force F of the extension of the rod is shown. The point P is the elastic limit.
The point P is the elastic limit.
Which shaded area represents the work done during the plastic deformation of the rod?

Question 9
A spring is suspended from a fixed point at one end. The spring is extended by a vertical force applied to the other end. The variation of the applied force with the length L of the spring is shown in figure a. State the name of the law that gives the relationship between the force and the extension.
a. State the name of the law that gives the relationship between the force and the extension.
b. Determine the spring constant, in N.m⁻¹.
c. Determine the elastic potential energy when F = 6 N.
Question 10
A spring is suspended from a fixed point at one end. A vertical force is applied to the other end, as shown in figure.
The variation of the applied force with the length L of the spring is shown in figure. a. Determine the spring constant of the spring.
a. Determine the spring constant of the spring.
b. Determine the elastic potential energy in the spring when the applied force is 15 N.